 So, the structure of chords is a topic that we will develop today. And, first of all, let's turn to the definition of a chord, clarify what it represents.
So, the structure of chords is a topic that we will develop today. And, first of all, let's turn to the definition of a chord, clarify what it represents.
Accord is a consonance, a sound complex. In a chord, at the same time or one after the other, at least three sounds should be performed in turn, because the harmonies in which only two sounds are called differently are intervals. And yet, the classical definition of a chord says that the sounds of a chord are either already located on the third line, or they can be arranged on the third line when rearranged. This last point is directly related to the structure of the chord.
Since modern harmony has gone far ahead of the norms established by the music of classical composers, this last remark about the arrangement of thrice sounds in a chord does not apply to some modern chords, since their structure is based on a different principle for constructing a chord. Such consonances appeared in which there can be three sounds or even more, but with all the desire, even if you try hard, you can’t arrange them for terts, but only, for example, by septima or seconds.
What is the structure of chords?
What does this all mean? First, it follows from this that the structure of the chords is their structure, the principle by which the tones (sounds) of the chord are located. Secondly, it also follows from the above that the structure of chords is of two types: tertsovy (classic version) and non-celtic (mainly characteristic of the music of the XX century, but met before). True, there is still so kind of chords with the so-called complicated teritz structure - with replaced, omitted or additional tones, but we will not consider this subspecies separately.
Tertsovy chords
With a tertz structure, chords are constructed from sounds located along the thirds. Different types of chords have such a structure: triads, seventh chords, non-chords along with their appeals. The figure shows just examples of such chords with tertzovoy structure - as Alexey Kofanov says, they somehow resemble snowmen.
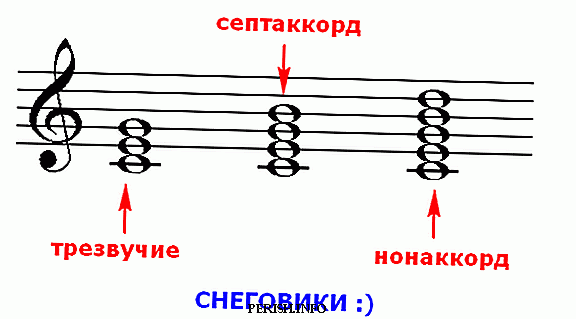
Now let's consider these chords as if under a magnifying glass. The structure of chords is formed by the intervals that make up a given chord (for example, the same third), and the intervals in turn consist of individual sounds, which are called “tones” of a chord.
The main sound of a chord is its base, the remaining tones will be called the same way as the intervals that these tones form with the base — that is, the third, fifth, septim, non, and so on. The names of all intervals, including the wide components, can be repeated using the materials on this page.
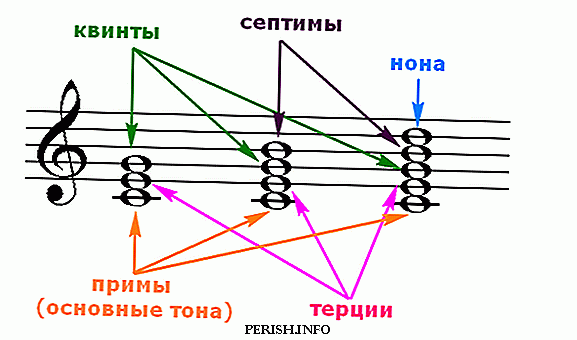
The structure of the chords is reflected in their name.
What is the need to determine the name of the tones in the chord? For example, in order for the structure of the chord to give it a name. For example, if a septima interval is formed between the base and the highest chord sound, then a chord is called a seventh chord, if a non is a nonaccord, if undecima, then, respectively, a undecimaccord. By analyzing the structure, you can give a name to any other chords, for example, all appeals of the dominant pendant.
So, in D7, in its basic form, all sounds are located along the thirds and between the base of the chord and its uppermost tone, a small septima interval is formed, which is why we call this chord a septa chord. However, in the appeals of D7 the arrangement of tones is different.
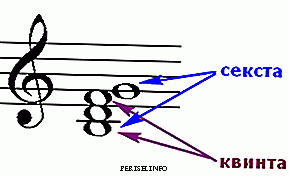 The first appeal of this seventh chord is the fifth-sixth chord. The name is given to it by the way the septima (upper tone D7) and the basic tone correspond to the chord bass, which intervals are formed. The basic tone in our example is the note salt, system, re - quit, and fa - septim. We see that the bass in this case is the note of the si, the distance from the note of the si to the note of the fa, which is septima is the fifth, and the note of the salt (the base of the chord) is the sixth. So it turns out that the name of the chord is made up of the names of two intervals - quints and seksta: fifth-sixth chord.
The first appeal of this seventh chord is the fifth-sixth chord. The name is given to it by the way the septima (upper tone D7) and the basic tone correspond to the chord bass, which intervals are formed. The basic tone in our example is the note salt, system, re - quit, and fa - septim. We see that the bass in this case is the note of the si, the distance from the note of the si to the note of the fa, which is septima is the fifth, and the note of the salt (the base of the chord) is the sixth. So it turns out that the name of the chord is made up of the names of two intervals - quints and seksta: fifth-sixth chord.
 Tertz-quart chord - where does its name come from? The bass chord in this example is the note re, everything else is still called. The distance from re to fa (septima) is termination, the interval from re to salt (base) is quart. Now everything is clear.
Tertz-quart chord - where does its name come from? The bass chord in this example is the note re, everything else is still called. The distance from re to fa (septima) is termination, the interval from re to salt (base) is quart. Now everything is clear.
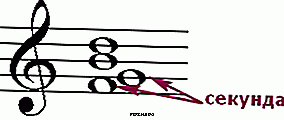 Now let's figure out the seconds with the chord. So, the bass note in this case is Mrs. Septima herself - note f. From fa to fa is prima, and the interval from the note fa to the bottom of the salt is second. The exact name of the chord should have been pronounced like a note-second chord. For some reason, the first root in this name is omitted, apparently, for convenience, and maybe because there is no interval between the septima and the septima - there is no repetition of the note fa.
Now let's figure out the seconds with the chord. So, the bass note in this case is Mrs. Septima herself - note f. From fa to fa is prima, and the interval from the note fa to the bottom of the salt is second. The exact name of the chord should have been pronounced like a note-second chord. For some reason, the first root in this name is omitted, apparently, for convenience, and maybe because there is no interval between the septima and the septima - there is no repetition of the note fa.
You can argue with me. How can these various fifths with second chords be attributed to tertiary chords? Indeed, there are other intervals in their structure than the third, for example, quarts or seconds. But here it is necessary to bear in mind that these chords are not nuggets in nature, these are just appeals of those snowman chords whose sounds feel great, being located along the third line.
Chords with non-tip structure
Yes, there are such too. For example, quart, quint consonances or the so-called "second clusters", try to arrange their sounds in third. I will just show you examples of such chords, and you can decide for yourself whether they are ordinary or not ordinary. See:

findings
Let's finally stop and summarize some of the results. We started by defining a chord. A chord is a consonance, a whole complex of sounds, in it there are at least three notes simultaneously or not at the same time, which are organized according to some structural principle.
We have named two types of chord structures: tertiary structure (typical for triads, septa chords with their appeals) and non-tercal structure (typical for second clusters, clusters, fifth, quart, and other chords). After analyzing the structure of the chord, you can give it a clear and precise name.

Leave Your Comment